Transforming Regular Grammars to Equivalent
Finite State Automata
Algorithm
The algorithm now follows:
- construct an
 -free regular grammar G' from G
(see relevant section);
-free regular grammar G' from G
(see relevant section);
- create a FSA M, with a state for every non-terminal in
G'. Set the state representing the start symbol to
be the start state;
- add another state D, which is terminal;
- if the production S

 is in G' (where S is
the start symbol of G', set the state representing
S to be final;
is in G' (where S is
the start symbol of G', set the state representing
S to be final;
- for every production A
 aB in G, add
a transition from state A to state B labelled
with terminal a;
aB in G, add
a transition from state A to state B labelled
with terminal a;
- for every production A
 a in G, add a
transition from A to the terminal state D
a in G, add a
transition from A to the terminal state D
Example
Construct a FSA M that recognises the language generated by
the following regular grammar G:
| S |  | a | aA | bB |  |
| A |  | aA | aS |
| B |  | cS |  |
where S is the start symbol.
- Construct an equivalent
 -free grammar:
-free grammar:
| S1 |  | a | b | aA | bB |  |
| S |  | a | b | aA | bB |
| A |  | a | aA | aS |
| B |  | c | cS |
where S1 is the start symbol.
- create a FSA M, with a state for every non-terminal in
G' - {S1, S, A, B}. S1,
being the start symbol is set to be the start state:

- add another terminsl state D0:

- since S1

 was in the grammar, state S1 is also set to
be final:
was in the grammar, state S1 is also set to
be final:

- for every rule of the form A
 aB, we add a transition
from state A to state B labelled a:
aB, we add a transition
from state A to state B labelled a:
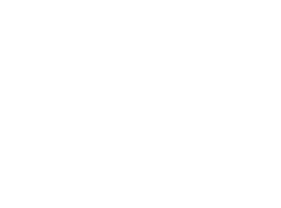
- for every rule of the form A
 a, we add a transition
from state A to state D0 labelled a:
a, we add a transition
from state A to state D0 labelled a:
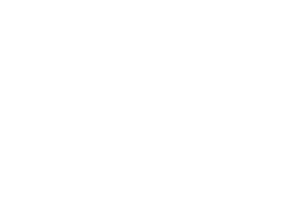
Exercises
Construct finite state automata equivalent to the following
regular grammars:
-
| G = < | { a, b, c }, |
| | { S, A, B, C }, |
| |
| { |
S |
 |
aA | bC, |
| |
A |
 |
aA | bB |
 , , |
| |
B |
 |
bB | b |
 , , |
| |
C |
 |
cB | cC |
 }, }, |
|
| | S > |
-
| G = < | { a, b, c }, |
| | { S, A, B, C }, |
| |
| { |
S |
 |
aA | bC |
 , , |
| |
A |
 |
aA | aS, |
| |
B |
 |
b, |
| |
C |
 |
cB | cS }, |
|
| | S > |
-
| G = < | { a, b, c }, |
| | { S, A, B, C }, |
| |
| { |
S |
 |
aA | bC |
 , , |
| |
A |
 |
aA | aS, |
| |
B |
 |
b, |
| |
C |
 |
cB | cS }, |
|
| | S > |
-
| G = < | { a, b }, |
| | { S, A }, |
| | |
| | S > |
-
| G = < | { a }, |
| | { S }, |
| | { S  }, },
|
| | S > |
 -free regular grammar G' from G
(see relevant section);
-free regular grammar G' from G
(see relevant section);

 is in G' (where S is
the start symbol of G', set the state representing
S to be final;
is in G' (where S is
the start symbol of G', set the state representing
S to be final;
 aB in G, add
a transition from state A to state B labelled
with terminal a;
aB in G, add
a transition from state A to state B labelled
with terminal a;
 a in G, add a
transition from A to the terminal state D
a in G, add a
transition from A to the terminal state D




